Mohrís Circle
Mohrís circle for plane stress:
Consider
the state of plane-stress shown in the figure.

If negative
the shear stress ![]() †is plotted against
the normal stress
†is plotted against
the normal stress ![]() †for each angle
†for each angle ![]() †of the incline, the
resulting plot will be a circle of radius R and with its center located at
†of the incline, the
resulting plot will be a circle of radius R and with its center located at ![]() . This can be directly shown by examining the equations for
. This can be directly shown by examining the equations for ![]() †and
†and ![]() .
.

This
circle is called Mohrís circle and is a powerful tool for visualizing the
possible states of stress at a material point. For example, at a glance one can
see that the maximum normal stress at the point is the average stress plus the
radius, the minimum normal stress at the point is the average stress minus the
radius and the maximum shear stress at the point is equal to the radius.

The equations to calculate these stresses are
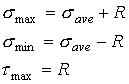
where

As can be seen the maximum and minimum normal stresses
occur on surfaces that have zero shear stress, but the maximum shear stress
occurs on a surface that has a normal stress equal to the average normal
stress.
The convention for positive and negative shear stress on
the Mohrís circle is given on the axis and in terms of the direction of the
shear stress on the stress element is shown in the following figure.

Locating points on Mohrís circle:
One
can locate points on Mohrís circle by locating the point representing the
surface normal to the x-axis, which has the coordinates ![]() , and then going along the circle an angle of
, and then going along the circle an angle of ![]() †to get to the state
of stress on the surface with an incline angle of
†to get to the state
of stress on the surface with an incline angle of ![]() .
.

On
Mohrís circle, point† X represent
the surface with normal along the x-direction and point Y
represents the surface with normal along the y-direction. Since these
surfaces are 90o apart on the stress element, on Mohrís circle they
are 180o apart (i.e., they end up on opposite sides of the
circle).†
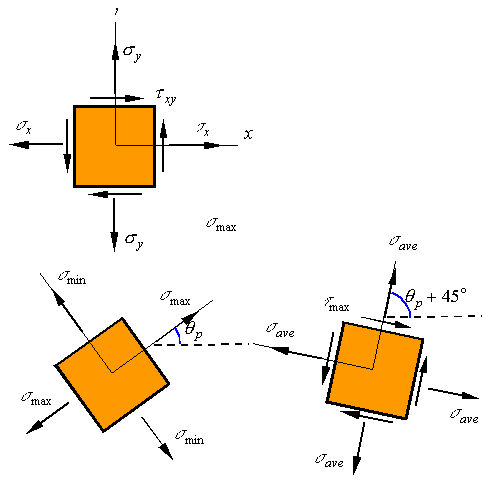
Principal stresses:
Principal
stresses referrer to the maximum and minimum normal stresses. As can be seen on
Mohrís circle, the principal normal stresses occur on surfaces which have no
shear stress. Also, the maximum shear stress is 90o away from the
maximum normal stress on Mohrís circle so that it is on a surface oriented 45o
away from the surface on which the maximum normal stress occurs.

Out of plane stresses:
Consider
the stress element shown where upon a state of plane stress a normal stress ![]() †is added in the third
direction. Since this added stress is along a direction perpendicular to the
plane stress, it does not interact with the in-plane stress (the stresses in
the x-y plane) and all the results obtained for plane stress still hold.
We can now rotate the stress element around the z-axis to get an element
along the principal directions (only normal stresses remain).
†is added in the third
direction. Since this added stress is along a direction perpendicular to the
plane stress, it does not interact with the in-plane stress (the stresses in
the x-y plane) and all the results obtained for plane stress still hold.
We can now rotate the stress element around the z-axis to get an element
along the principal directions (only normal stresses remain).

Each
two of the resulting directions now represents a simple state of plane stress
for which shear stresses are zero. One can draw the three Mohrís circles which
result from each pair of directions to get the following Mohrís circles. One
can show that all states of stress fall between the three circles (the shaded
area).

The
absolute maximum and minimum normal stresses depend on the relative values of
the three principal stresses ![]() ,
, ![]() , and
, and ![]() †(and need not be as
shown in the figure). Also the maximum shear stress will equal the radius of
the largest circle.
†(and need not be as
shown in the figure). Also the maximum shear stress will equal the radius of
the largest circle.
![]()
„ Mehrdad Negahban and the University of Nebraska, 1996-2000.
All rights reserved
Copy and distribute freely for personal use only
Department of Engineering Mechanics, University of Nebraska, Lincoln, NE 68588-0526
Last modified at: 9:42
AM,
Monday, March 13, 2000